A Sharpened Condition for Strict Log-Convexity of the Spectral Radius

A Sharpened Condition for Strict Log-Convexity of the Spectral Radius via the Bipartite Graph

Lee Altenberg

2013. Linear Algebra and Its Applications 438 (9): 3702-3718. Submitted August 5, 2012.

Abstract

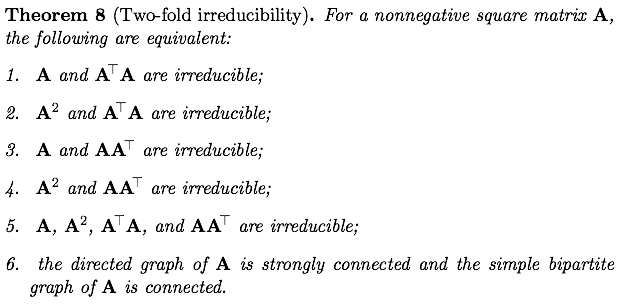
Friedland (1981) showed that for a nonnegative square matrix A, the spectral radius r(e^D A) is a log-convex functional over the real diagonal matrices D, and that when A is fully indecomposable, log r(e^D A) is strictly convex over a convex combination of D₁, D₂ if and only if D₁ - D₂ ≠ c I for any c ∈ R. Here the condition of full indecomposability is shown to be replaceable by the weaker condition that A and A^TA be irreducible, which is the sharpest possible replacement condition. Irreducibility of both A and A^TA is shown to be equivalent to irreducibility of A² and A^TA, which is the condition for a number of strict inequalities on the spectral radius found in Cohen, Friedland, Kato, and Kelly (1982). Such ‘two-fold irreducibility’ is equivalent to joint irreducibility of A, A², A^TA, and A A^T, or in combinatorial terms, equivalent to the directed graph of A being strongly connected and the simple bipartite graph of A being connected. Additional ancillary results are presented.

Lee Altenberg's Home Page | Papers | E-mail me