On the Ordering of the Spectral Radius Product r(A)r(AD) Versus r(A2D) and Related Applications
Lee Altenberg
SIAM Journal on Matrix Analysis and Applications 34(3): 978-998 (2013). Submitted January 16, 2013.
Abstract
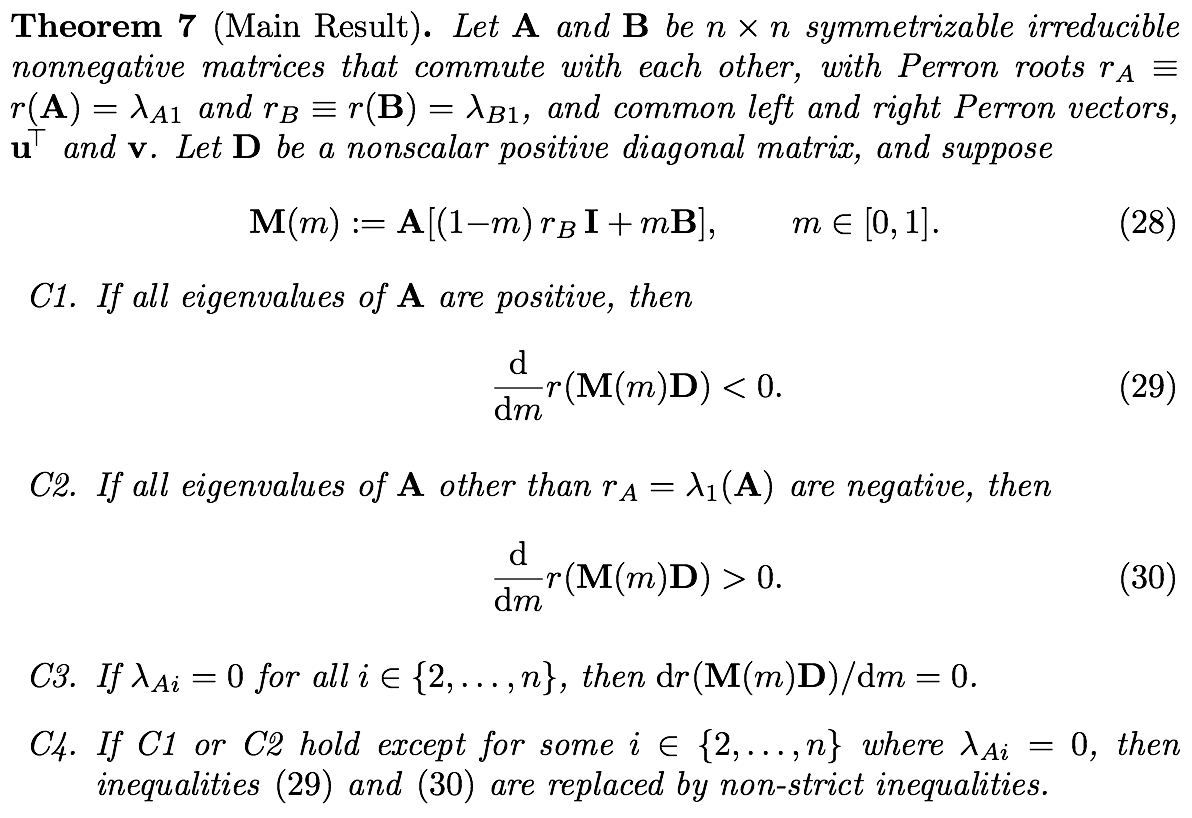
For a nonnegative matrix A and real diagonal matrix D, two known inequalities on the spectral radius, r(A2D2) ≥ r(AD)2 and r(A)r(A D2) ≥ r(AD)2, leave open the question of what determines the order of r(A2D2) with respect to r(A)r(A D2). This is a special case of a broad class of problems that arise repeatedly in ecological and evolutionary dynamics. Here, sufficient conditions are found on A that determine orders in either direction. For a symmetrizable nonnegative matrix A with all positive eigenvalues and nonnegative D, r(A2D) ≤ r(A)r(AD). The reverse holds if all of the eigenvalues of A are negative besides the Perron root. This is a particular case of the more general result that r(A [(1-m)r(B)I + mB] D) is monotonic in m when all non-Perron eigenvalues have the same sign — decreasing for positive signs and increasing for negative signs, for symmetrizable nonnegative A and B that commute. Commuting matrices include the Kronecker products A, B ∈ { ⊗i=1 to L Miti }, ti ∈ {0, 1, 2, ...}, which comprise a class of application for these results. This machinery allows analysis of the sign of ∂/∂mj r({⊗i=1 to L [(1-mi)r(Ai) Ii + mi Ai] }D). The eigenvalue sign conditions also provide lower or upper bounds to the harmonic mean of the expected sojourn times of Markov chains. These inequalities appear in the asymptotic growth rates of viral quasispecies, models for the evolution of dispersal in random environments, and the evolution of site-specific mutation rates over the entire genome.
Lee Altenberg's Home Page |
Papers |
E-mail me

