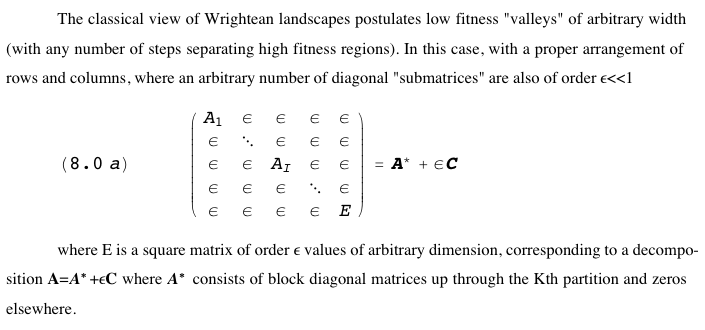
|

|
In this paper, we investigate fitness landscapes (under point mutation and recombination) from the standpoint of whether the induced evolutionary dynamics have a "fast-slow " time scale associated with the differences in relaxation time between local quasi-equilibria and the global equilibrium. This dynamical behavior has been formally described in the econometrics literature in terms of the spectral properties of the appropriate operator matrices by Simon and Ando (1961), and we use the relations they derive to ask which fitness functions and mutation/recombination operators satisfy these properties. It turns out that quite a wide range of landscapes satisfy the condition (at least trivially) under point mutation given a sufficiently low mutation rate, while the property appears to be difficult to satisfy under genetic recombination. In spite of the fact that Simon-Ando decomposability can be realized over fairly wide range of parameters, it imposes a number of restrictions on which landscape partitionings are possible. For these reasons, the Simon-Ando formalism does not appear to be applicable to other forms of decomposition and aggregation of variables that are important in evolutionary systems.
Keywords: Fitness Landscapes, Aggregation of Variables, Decomposability, Mutation, Selection, Dynamical Systems
Citations: Simon, Herbert A. and A. Ando (1961). Aggregation of variables in dynamic systems. Econometrica: 29:111-138.